Area Of A Circle Formula Using Radius

In technical terms, a circle is a locus of a point moving around a fixed point at a fixed distance away from the point. Basically, a circleis a closed curve with its outer line equidistant from the center. The fixed distance from the point is the radius of the circle. In real life, you will get many examples of the circle such as a wheel, pizzas, a circular ground, etc.

Now let us learn, what are the terms used in the case of a circle. A circle is a collection of points that are at a fixed distance from the center of the circle. We see circles in everyday life such as a wheel, pizzas, a circular ground, etc. The measure of the space or region enclosed inside the circle is known as the area of the circle.

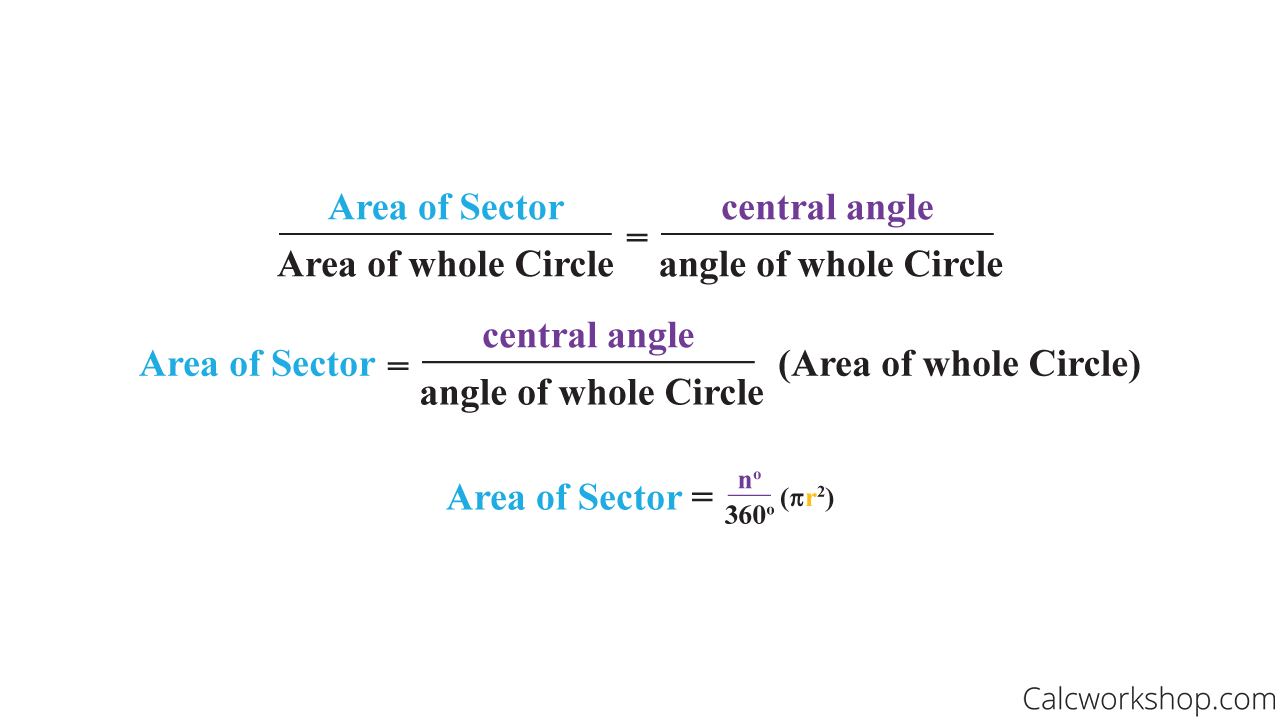
It is easy to go from the area of a triangle to the area of a regular polygon by breaking the polygon into triangles and summing the areas of the triangles. But we need to first review the formula for the area of a polygon for reference. It can be shown that every regular polygon can be inscribed in a circle. If we draw a perpendicular line from the center of the circle to any side of the inscribed polygon, that line is called an apothem. It is a fact that the area of a regular polygon is $1/2ap$ where $a$ is the apothem and $p$ is the perimeter of the polygon. The distance from the center to any point on the circle is called its radius.

Any line segment, the endpoints of which lie on the circle and it passes through the center is the diameter of the circle. With the help of a radius, we can find the area of circle. In our surroundings, the most common circle shape would be of the moon and planets. Long ago, the shape of a circle inspired the creation of a wheel which serves as the basis for most of the machinery in the modern world. Geometry, astronomy, and calculus all involve the study of circles.

The surface is represented in square units. The circle is divided into 16 equal sectors, and the sectors are arranged as shown in fig. The area of the circle will be equal to that of the parallelogram-shaped figure formed by the sectors cut out from the circle. Since the sectors have equal area, each sector will have an equal arc length. The red coloured sectors will contribute to half of the circumference, and blue coloured sectors will contribute to the other half. Since area is a measure of two dimensions, you always report area in square units like square inches or square feet .

The area of the circle can be conveniently calculated either from the radius, diameter, or circumference of the circle. The constant used in the calculation of the area of a circle is pi, and it has a fractional numeric value of 22/7 or a decimal value of 3.14. Any of the values of pi can be used based on the requirement and the need of the equations. The below table shows the list of formulae if we know the radius, the diameter, or the circumference of a circle. The area of a circle formula is useful for measuring the region occupied by a circular field or a plot.

Suppose, if you have a circular table, then the area formula will help us to know how much cloth is needed to cover it completely. The area formula will also help us to know the boundary length i.e., the circumference of the circle. A circle is a two-dimensional shape, it does not have volume. A circle only has an area and perimeter/circumference. Let us learn in detail about the area of a circle, surface area, and its circumference with examples.

A circle is a closed curve formed by a set of points on a plane that are the same distance from its center. The area of a circle is the region enclosed by the circle. The area of a circle is equals to pi (π) multiplied by its radius squared. For those having difficulty using formulas manually to find the area, circumference, radius and diameter of a circle, this circle calculator is just for you. The equations will be given below so you can see how the calculator obtains the values, but all you have to do is input the basic information. In this method, we divide the circle into 16 equal sectors.

The sectors are arranged in such a way that they form a rectangle. All sectors are similar in area, so hence all sectors' arc length would be equal. The circle's area would be the same as the area of the parallelogram shape or rectangle. This surface area of the circle is represented in terms of square units.

A circle is known as a closed plane geometrical shape. Technically, it is the locus of a point that moves around a fixed point at a fixed distance that is away from that point. A circle is basically a closed curve that has its outer line at an equal distance from the centre. This fixed distance from the central point is known as the radius of the circle. In our day to day life, we often see many examples like a pizza, wheel, etc.

Let us learn about these terms in regards to a circle. This area is the region that occupies the shape in a two-dimensional plane. Now we will learn about the area of the circle. So the area covered by one complete cycle of the radius of the circle on a two-dimensional plane is the area of that circle. Now how can we calculate the area for any circular object or space? In this case, we use the formula for the circle's area.

A circle can be divided into many small sectors which can then be rearranged accordingly to form a parallelogram. When the circle is divided into even smaller sectors, it gradually becomes the shape of a rectangle. We can clearly see that one of the sides of the rectangle will be the radius and the other will be half the length of the circumference, i.e, π. As we know that the area of a rectangle is its length multiplied by the breadth which is π multiplied to 'r'.

Only a mathematician can genuinely understand the practical importance of formulas for calculating area, radius, diameter, or circle circumference. While most people think that formulas have no practical use, they are critical factors in many everyday life routines. The perimeter of a closed figure is known to be the length of its total boundary.

When it comes to the circles, the perimeter is called by a different name. It is referred to as the 'circumference' of the given circle. This circumference is known as the total length of the boundary of the given circle. If we open the circle and form a straight line, the length of the straight line that we get is the circumference. For defining the circumference of a circle, we need to know a term called 'pi'.

Consider the circle shown below having its centre at O and radius r. We use this formula to measure the space which is occupied by either a circular plot or a field. In this article today, we will discuss the area of circle definition, the area of a circle equation, its circumference and surface area in detail. The area of a circle can be thought of as the number of square units of space the circle occupies. This can be found using either the radius or the diameter, which we will cover in the examples below.

We will also look at some examples of word problems involving area that you may come across in your studies. The perimeter of circle is nothing but the circumference, which is equal to twice of product of pi (π) and radius of circle, i.e., 2πr. We have discussed till now the different parameters of the circle such as area, perimeter or circumference, radius and diameter. Let us solve some problems based on these formulas to understand the concept of area and perimeter in a better way. As we know, the area of circle is equal to pi times square of its radius, i.e. π x r2.

To find the area of circle we have to know the radius or diameter of the circle. Fill the circle with radius r with concentric circles. After cutting the circle along the indicated line in fig. 4 and spreading the lines, the result will be a triangle. The base of the triangle will be equal to the circumference of the circle, and its height will be equal to the radius of the circle.

A perimeter of closed figures is defined as the length of its boundary. When it comes to circles, the perimeter is given using a different name. It is called the "Circumference" of the circle. This circumference is the length of the boundary of the circle.

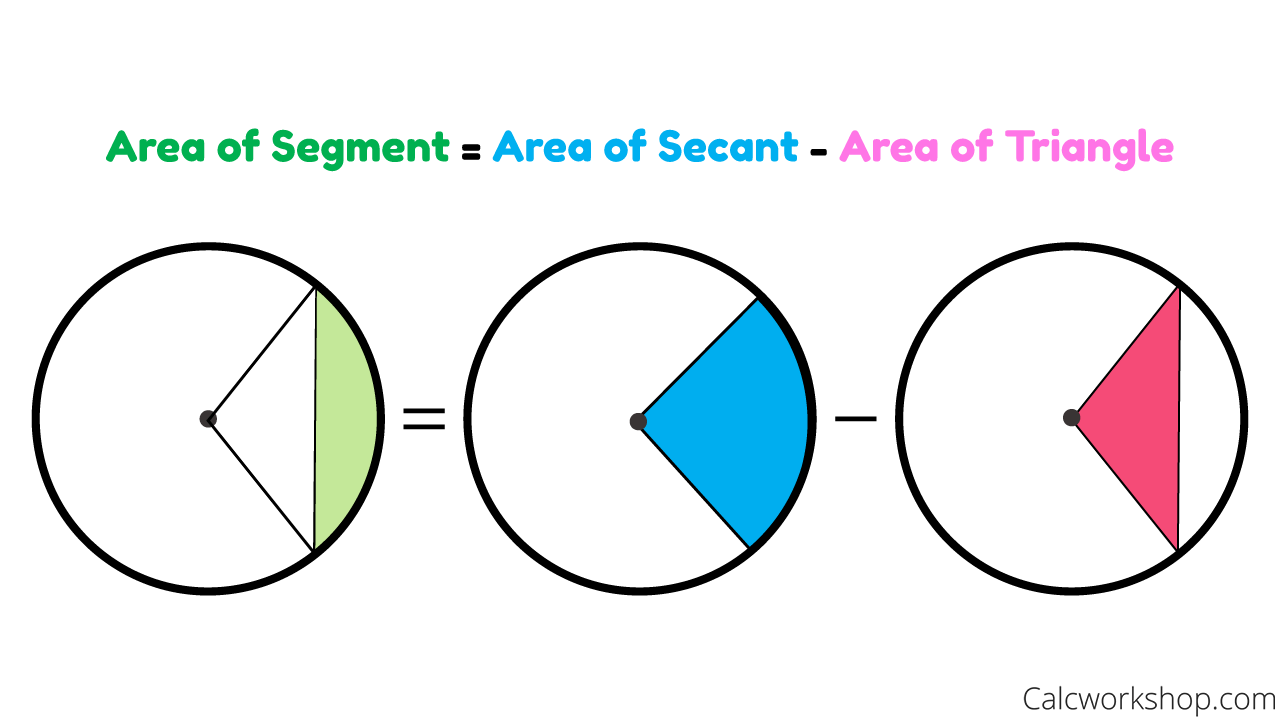
If we open the circle to form a straight line, then the length of the straight line is the circumference. To define the circumference of the circle, knowledge of a term known as 'pi' is required. If the area of the circle is not equal to that of the triangle, then it must be either greater or less. We eliminate each of these by contradiction, leaving equality as the only possibility.

The area of a circle is the amount of space enclosed within the boundary of a circle. The region within the boundary of the circle is the area occupied by the circle. It may also be referred to as the total number of square units inside that circle.

The area A is equal to pi times the diameter divided by 2, squared. Thus, the area of a circle A is equal to pi times the radius squared. It involves dividing the circle into many sectors and rearranging the sectors to form a rectangle. The base of the rectangle is shown to be πr and the height of the rectangle is r. The area of the rectangle is then the product of πr and r.

The area of the circle which is equal to area of the rectangle is then πr2. Finding the radius is not always easy, especially if you don't have the circle's center. You can calculate the area using the diameter instead. The same formula applies as above, but you need first to calculate the radius of the circle. Simply divide the diameter by 2 to get the radius. In this image, you can see 16 sectors, including 8 green and 8 blue ones.

The green highlighted sectors represent the circle's half circumference while the other half of circumference is represented by blur highlighted ones. By increasing the number of the sectors cut from the circle, the parallelogram will change into a rectangle. The length of the rectangle would b equal to πr with a width equal to r. The area of a circle is any space that the circle occupies on a flat surface.

When we talk about the surface area of the circle, we are focusing on two-dimensional objects. When finding the circle area, there are three other measures that we take into consideration, including the circumference, diameter, and radius. All three calculations also help us fining the circle area. It's worth noting that the area of a circle, like the area of every other number, is calculated in square units. So, if you have a circular radius, square the length of the radius and multiply it by the value of pi to get the area of the square. The perimeter, as we know, is the path that surrounds a shape.

In the case of a circle, the perimeter is defined as the circumference of the circle. Pi is a number that is used to describe the circumference to diameter ratio of a circle. Pi's value remains constant regardless of the size of the circle. A circle is defined as the shape consisting of all points in a plane that are at an equal distance from a given point called the center. Alternatively, it is the curve traced out by a point moving in a plane so that its distance from a given point is constant. This center is known as the circle's middle point.

If the diameter of a circle is known then the formula for radius is diameter divided by 2. If the circumference of a circle is known then the formula for radius is the circumference divided by 2 pi. And if the area of a circle is known then the formula for radius is the square root of the area divided by pi.

Hence, the concept of area as well as the perimeter is introduced in Maths, to figure out such scenarios. But, one common question that arises among most people is "does a circle have volume? Since a circle is a two-dimensional shape, it does not have volume. In this article, let us discuss in detail the area of a circle, surface area and its circumference with examples. Term Definition Area Area is the space within the perimeter of a two-dimensional figure. Circle A circle is the set of all points at a specific distance from a given point in two dimensions.

Diameter Diameter is the measure of the distance across the center of a circle. The diameter is equal to twice the measure of the radius. The area of the circle is the measure of the space or region enclosed inside the circle. In simple words, the area of a circle is the total number of square units inside that circle. The answer will be square units of the linear units, such as mm2, cm2, m2, square inches, square feet, and so on. In some real world situations, you may not be able to measure the diameter or radius accurately.

If the diameter is not drawn for you or the center is not identified, it can be difficult to approximate the center of a circle. Move the loose number over to the other side. Divide off by whatever is multiplied on the squared terms. Take the coefficient on the x-term, multiply by one-half, square, and add inside the x-stuff and also to the other side. Convert the left-hand side to squared form, and simplify the right-hand side. If necessary, fiddle with signs and exponents to make your equation match the circle equation's format.

The center is at ( 1/2, – 6/5 ) and the radius is 3/2. A circle is a closed shape formed by tracing a point that moves in a plane such that its distance from a given point is constant. The word circle is derived from the Greek word kirkos, meaning hoop or ring.